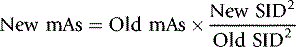Chapter 9 Production of X-rays
 The kinetic energy of projectile electrons transfers to target atoms. Approximately 99% of the energy converts into heat and only about 1% converts into X-rays.
The kinetic energy of projectile electrons transfers to target atoms. Approximately 99% of the energy converts into heat and only about 1% converts into X-rays. A bremsstrahlung interaction involves projectile electrons that emit radiation as they slow down when passing close to the nucleus of target atoms.
A bremsstrahlung interaction involves projectile electrons that emit radiation as they slow down when passing close to the nucleus of target atoms. A characteristic interaction involves the emission of radiation following a collision between projectile electrons and the orbital electrons of target atoms.
A characteristic interaction involves the emission of radiation following a collision between projectile electrons and the orbital electrons of target atoms. X-ray beam quality and quantity are affected by the target material, beam filtration, distance, and prime exposure factors (kVp, mA, and exposure time).
X-ray beam quality and quantity are affected by the target material, beam filtration, distance, and prime exposure factors (kVp, mA, and exposure time). The target material affects both the quality and quantity of the X-ray beam. Tungsten is the standard target material due to its high proton number.
The target material affects both the quality and quantity of the X-ray beam. Tungsten is the standard target material due to its high proton number. Peak kilovoltage (kVp) controls the quality of the beam. As kVp increases, X-rays are more penetrating and as kVp decreases, X-rays become less penetrating.
Peak kilovoltage (kVp) controls the quality of the beam. As kVp increases, X-rays are more penetrating and as kVp decreases, X-rays become less penetrating. kVp affects the quantity of X-rays produced. An increase in kVp rapidly increases the number of X-ray photons and a decrease in kVp rapidly decreases the number of X-rays. The amount of radiation produced is proportional to the square of the ratio of the kVp.
kVp affects the quantity of X-rays produced. An increase in kVp rapidly increases the number of X-ray photons and a decrease in kVp rapidly decreases the number of X-rays. The amount of radiation produced is proportional to the square of the ratio of the kVp. 15% rule: a 15% increase in kVp doubles radiographic density; a 15% decrease in kVp halves radiographic density.
15% rule: a 15% increase in kVp doubles radiographic density; a 15% decrease in kVp halves radiographic density. Reciprocity law: any combinations of mA and time that give the same mA s will result in the same quantity of X-ray photons.
Reciprocity law: any combinations of mA and time that give the same mA s will result in the same quantity of X-ray photons.INTRODUCTION
Diagnostic X-rays are produced in the target of the anode when high-energy projectile electrons are rapidly decelerated. Diagnostic X-ray imaging equipment provides the means for practitioners to control the quality and quantity of the X-ray beam. Consequently, it is important to understand the process of X-ray production and the factors that influence the characteristics of the beam. Practitioners familiar with the concepts and factors that influence quality and quantity are better able to control exposure factors to produce optimal radiographic images while minimizing patient dose.
ELECTRON PRODUCTION
Four conditions are necessary for the production of diagnostic X-rays:
In the X-ray tube, the purpose of the filament is to provide the free electrons necessary for X-ray production. As the rotor is activated the current passing through the filament heats to the point where electrons boil off. This process is referred to as thermionic emission. At this point, a space charge (cloud of electrons) forms around the filament. The focussing cup temporarily concentrates the free electrons and helps form them into a beam.
When the exposure begins, the primary circuit closes and a high voltage is applied across the anode (positively charged) and cathode (negatively charged). This causes electrons to stream towards the anode at a high rate of speed. The potential energy of each electron is one kiloelectron volt (keV) of energy for each kilovolt (kV) of voltage set for the exposure. Electrons (sometimes called projectile electrons) that travel from the cathode to anode make up the tube current.
TARGET INTERACTIONS
When the high-speed projectile electrons collide with the X-ray tube target they interact with the orbital electrons or the nuclear field of the target atoms. Kinetic energy transferred from the projectile electrons to the target atoms converts into heat or X-rays. When projectile electrons strike outer target shell electrons it puts them in an excited state and as a result, infrared (heat) radiation is emitted. Approximately 99% of the energy of projectile electrons converts into heat. Only about 1% of the energy converts into X-ray photons. Two types of interaction produce X-ray photons: bremsstrahlung interactions and characteristic interactions.
BREMSSTRAHLUNG INTERACTIONS
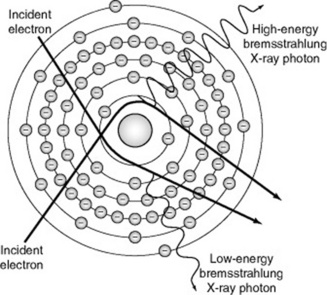
Bremsstrahlung in German means ‘to brake radiation’ or braking radiation. Bremsstrahlung target interaction occurs when projectile electrons pass by outer shell electrons of target atoms and interact with the force field of the nucleus of the atom. Because atomic nuclei are positively charged and electrons are negatively charged, there is a mutual attraction between them. The nuclear force field causes the entering electron to slow down (or brake) and change direction. The loss of kinetic energy that occurs when a projectile electron slows down is emitted as an X-ray photon. These X-ray photons are known as bremsstrahlung photons or brems radiation (Fig. 9.1). In the diagnostic range, approximately 85% of X-ray emissions are the result of bremsstrahlung interactions.
CHARACTERISTIC INTERACTIONS
Characteristic target interaction occurs when projectile electrons interact with inner shell electrons of target atoms. Recall that orbital electrons within an atom have a specific binding energy. The binding energy, based on the size of the atom and the shell in which the electron is located, is the energy that would be required to remove the electron from the atom.
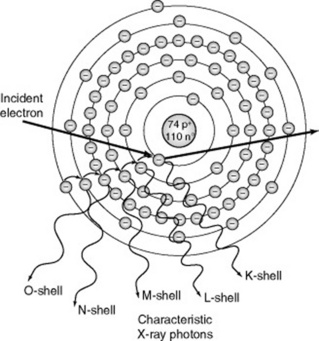
Characteristic radiation is produced when projectile electrons with sufficient kinetic energy eject an inner orbital electron (Fig. 9.2). When this happens, the atom becomes unstable and temporarily ionised because of the missing electron. An electron from an outer shell instantly fills the void created by the missing electron and an X-ray photon is emitted. This process continues until the atom is stable. The energy of the emitted X-ray photon is equal to the difference between the binding energy of the two involved orbital electrons. Accordingly, each X-ray photon has a specific energy level. This explains why this type of emission is called characteristic radiation. The energy emitted is characteristic of the target element and the involved shells.
Higher energy X-ray photons result with target materials of a higher proton number and interactions that involve the ejection of inner shell electrons. Each target element emits characteristic radiation of a given energy. For example, the K shell binding energy for tungsten is 69.5 keV. Only projectile electrons with energies greater than the K shell binding energy are able to eject K shell electrons. Accordingly, K shell characteristic X-rays are only produced when the applied voltage exceeds 69.5 kVp. In comparison, the characteristic radiation of a molybdenum target (often used for mammography) is very different. The K shell binding energy for molybdenum is 20 keV,so K shell characteristic X-rays are produced when the applied voltage exceeds 20 kVp.
With a tungsten target, only K shell interactions result in X-rays of sufficient energy to be beneficial in diagnostic radiography. All other characteristic radiation has very low energy and falls outside the useful diagnostic range. Approximately 15% of X-ray emissions are the result of characteristic interactions.
EMISSION SPECTRUM
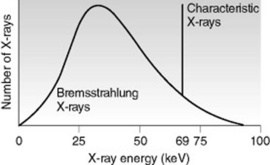
The emission spectrum is a graphic representation of the number of X-rays plotted against the energy of the radiation, which is measured in kiloelectron volts (keV) (Fig. 9.3). The emission spectrum for bremsstrahlung radiation is continuous because bremsstrahlung X-rays include a range of energies. The emission spectrum for characteristic radiation is discrete because characteristic X-rays consist of predictable energies that are specific to the target element.
CONTINUOUS X-RAY SPECTRUM
Bremsstrahlung radiation is graphically illustrated as a continuous spectrum. The energy of a bremsstrahlung photon is the difference between the entering and exiting kinetic energy of the projectile electron. As a result, there is a continuous range of X-ray energies from zero to the maximum established by the potential difference across the X-ray tube. Maximum energy is realised if all the kinetic energy of an electron is converted into a singleX-ray photon. The maximum photon energy, determined by the maximum voltage, is the kilovolt peak (kVp). For example, if the potential difference across the X-ray tube were 90 kVp, an electron accelerated across the tube would attain a kinetic energy of 90 keV as it interacted with the target. If the electron transferred all of its energy, the energy of the X-ray photon would be 90 keV. The maximum photon energy is dependent on the potential difference across the tube (kVp), regardless of the target material.
The size and shape of the emission spectrum reflects the quality and quantity of the X-ray beam. While the relative shape of the emission spectrum remains the same, its location along the horizontal axis can vary. Ranges located more towards the right represent X-ray beams of higher energy or quality. Graphically, the area under the curve represents the total number of X-rays emitted. A larger area represents X-ray beams with higher intensity or quantity. The greatest number of X-rays have approximately one-third to one-half of the maximum energy.1
DISCRETE X-RAY SPECTRUM
Characteristic radiation is graphically illustrated in the form of a line spectrum. Remember that the energy of a characteristic photon depends on the differences between the electron binding energies of a particular target material. As a result, the spectrum produced by characteristic X-rays is referred to as discrete or distinct. For example, there are only 15 specific energy levels of characteristic X-rays from tungsten: five from interactions at the K shell, four from interactions at the L shell, and the remainder from interactions at lower energy outer shells. In tungsten, only characteristic X-rays produced from the five K shell interactions are of sufficient energy to be of diagnostic value. The number of photons produced at each characteristic energy level is different because the likelihood for filling a K shell void varies from shell to shell. Often, the five energy levels are represented on the emission spectrum as a single line. As illustrated in Figure 9.3, the vertical line at 69 keV represents the characteristic K X-rays of tungsten.
X-RAY QUALITY AND QUANTITY
The quality of radiation in an X-ray beam is the penetrating ability of the beam. The quantity of radiation in an X-ray beam is the number of photons in the beam. The terms exposure and intensity may also be used to describe quantity.
While practitioners have little control over the selection of the target material and limited options for the use of added beam filtration, it is valuable to understand how the target material and beam filtration affect the quality and quantity of the X-ray beam. Practitioners are able to control distance and prime exposure factors. Consequently, it is essential to understand how these factors influence the quality and quantity of the X-ray beam (Table 9.1).
Table 9.1 Summary of factors affecting X-ray quality and quantity
| Factors affecting X-ray quality | Factors affecting X-ray quantity |
|---|---|
| Target material | Target material |
| Beam filtration | Beam filtration |
| kVp | Distance |
| kVp | |
| mA s |
kVp, kilovolt (peak); mA s, milliamp-second
TARGET MATERIAL
The proton number of the target material affects both the quality and quantity of the X-ray beam. As mentioned previously, tungsten is the chief target material used in diagnostic radiography due to its high proton number (Z) of 74. A target material with a higher proton number results in increased production of bremsstrahlung radiation. Bremsstrahlung production is also more efficient because more high energy X-rays are produced relative to low energy X-rays. A target material with a higher proton number also results in the production of characteristic radiation of higher energy. In contrast, the molybdenum (Z = 42) and rhodium (Z = 45) targets used for mammography have much lower proton numbers. These targets produce the lower energy radiation necessary for this imaging application.
BEAM FILTRATION
Filtration of the X-ray beam affects both the quality and quantity. Beam filtration changes the characteristics of the beam by removing ineffective low energy X-rays. Inherent and added filtration reduces the quantity and increases the average energy of the X-ray beam. The result is reduced patient skin dose.
DISTANCE
The distance of the anode from the image receptor (source–image distance, SID) affects the quantity of X-rays photons (see p. 91). The inverse square law governs the relationship between the quantity of X-ray photons and the distance from the target to the image receptor. The quantity of X-ray photons at the image receptor is inversely proportional to the square of the distance from the source (see p. 91). For example, if the SID is reduced by one-half, the number of X-ray photons quadruples.
PRIME EXPOSURE FACTORS
The prime exposure factors include kVp, mA, and exposure time. The kVp affects both the quality and quantity, while mA and exposure time affect the quantity of the X-ray beam.
Kilovoltage (kVp)
The kilovoltage peak (kVp) set by the practitioner determines the voltage or potential difference applied across the cathode and anode during the exposure. This setting affects both the quality and quantity of the X-ray beam. As mentioned earlier, the kVp setting controls the speed of the electrons travelling from the cathode to the anode. An increase in kVp causes greater repulsion of electrons from the cathode and greater attraction of electrons towards the anode. This increased speed means projectile electrons possess greater potential energy.
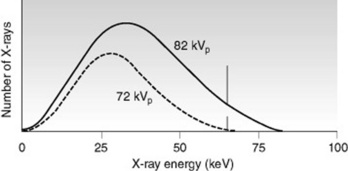
Changes in kVp affect the production of bremsstrahlung radiation, which influences both the quality and quantity of photons in the X-ray beam. An increase in kVp results in higher quality X-ray photons with a higher average energy and more penetrating ability. Keep in mind that the maximum energy of an X-ray beam remains equal to the kVp setting. With an increase in kVp there is also an increase in the quantity of X-ray photons at all energy levels. However, the increase is relatively greater for high energy X-rays than for low energy X-rays. The emission spectrum in Figure 9.4 illustrates how the area under the curve increases and shifts to the right as kVp is increased.
Changes in kVp also affect the production of characteristic radiation, which influences the quantity but not the quality of photons in the X-ray beam. Recall that no characteristic radiation is produced if the kVp is less than the binding energy of the K shell electrons. For example, no characteristic radiation is produced when the applied voltage is less than 69.5 kVp for a tungsten target because the binding energy of the K shell is 69.5 keV. However, the quantity of characteristic radiation increases when the kVp exceeds the K shell binding energy. The increase is typically proportional to the difference between the kVp and the binding energy.
Milliamperage (mA) and exposure time
The milliamperage (mA) set by the practitioner determines the quantity of electrons in the tube current. The relationship between mA and the quantity of X-ray photons produced is directly proportional. As mA is increased, the quantity of electrons in the tube current and the number of X-ray photons increases proportionally. As mA is decreased, the quantity of electrons in the tube current and the number of X-ray photons decreases proportionally.
The exposure time set by the practitioner controls the length of time electrons are permitted to travel from the cathode to the anode. The relationship between exposure time and the quantity of X-ray photons produced is directly proportional. As exposure time is increased, the quantity of electrons and the number of X-ray photons increases proportionally. As exposure time is decreased, the quantity of electrons and the number of X-ray photons decreases proportionally.
In sum, the quantity of electrons that travel from the cathode to the anode and the quantity of X-ray photons produced are directly proportional to the mA and exposure time. The milliampere-second (mA s) is the product of mA and exposure time. The mA s affects only the quantity of photons in the X-ray beam; it does not affect the quality or energy of the X-ray photons.
EXPOSURE MANIPULATION
Exposure manipulation includes those variables that practitioners most often employ to manage the quality and quantity of the X-ray beam. Distance, kVp, and mA s are the primary factors considered here.
DISTANCE
As stated previously, the inverse square law governs the relationship between X-ray quantity and distance. The formula for the inverse square law is effective when the intensity of the exposure in sieverts is known. A practical alternative is to manipulate mA s to compensate for changes in distance. The square law (a derivative of the inverse square law) calls for a change in mA s by the factor of SID2.
PEAK KILOVOLTAGE (kVp)
The kVp controls the energy or quality of the X-rays produced at the anode and as such determines the penetrating ability of the X-rays produced. As kVp increases, X-rays are more penetrating and as kVp decreases, X-rays become less penetrating. The penetrating ability of X-rays also has some bearing on the number of X-rays exiting the patient. If the penetrating ability of X-ray photons is insufficient, the quantity of photons is irrelevant. In other words, increased mA s cannot compensate for inadequate kVp.
kVp also affects the quantity of radiation. As kVp increases, the number of X-ray photons rapidly increases, and as kVp decreases, the number of X-ray photons rapidly decreases. Unlike with mA s the relationship is not directly proportional. The amount of radiation produced is proportional to the square of the ratio of the kVp. For example, if kVp is doubled, the number of X-ray photons quadruples.
In practice, using kVp to manage the quantity of X-ray photons is unrealistic. Again, because kVp affects the penetrating ability of the X-ray beam, it is important to select the optimal kVp for a given part. However, when it is necessary to vary kVp the 15% rule is helpful. The 15% rule states that a 15% increase in kVp will double radiographic density, whereas a 15% decrease in kVp will halve radiographic density.
MILLIAMPERE-SECOND (mA s)
Milliampere-seconds controls the number of electrons passing from the cathode to the anode and as such controls the quantity of X-ray photons produced at the anode. There is a directly proportional relationship between the quantity of X-ray photons produced and the mA s. In other words, as mA s is increased, the number of X-ray photons increases by the same proportion and as mA s is decreased, the number of X-ray photons decreases proportionally. The law of reciprocity states that any combinations of mA and time that give the same mA s will produce the same number of X-ray photons.
Bushong S. Radiologic science for technologists: physics, biology, and protection, 8th edn, St Louis: Mosby; 2004:147-160.
This provides clear descriptions on the production of X-rays, with good diagrams..
Fauber TL. Radiographic imaging and exposure, 2nd edn, Mosby: St Louis; 2004:19-32.
This text gives clear descriptions with practical tips on the effects of exposure on a radiographic image, with images to support the written content..